The Fundamental Interactions between X-rays and Matter

X-radiation is a form of electromagnetic radiation, the wavelength of which is in the range of 0.05 to 10 nanometres. X-rays with wavelengths longer than 0.1 nm are classified as soft X-rays, while those with shorter wavelengths are known as hard X-rays. The wavelength range of the gamma rays partly overlaps with that of the X-rays. The two types of radiation are distinguished by the source: X-rays are created by interactions between electrons and atoms or between charged particles and an electromagnetic field, while gamma radiation is the electromagnetic radiation generated during nuclear transformations. During the electromagnetic interaction between the atoms and the electrons the electrons slow down and X-rays are emitted. If the electron which collides with the atom has a sufficiently high kinetic energy, it can knock out one of the electrons of the atom and the electron deficiency can be filled by a higher-energy electron of the atom. During this process the atom emits an X-ray photon, the so-called characteristic X-ray. X-radiation was discovered by the German physicist Wilhelm Conrad Röntgen, who won the Nobel Prize for his discovery in 1901.
X-rays are electromagnetic waves, so similarly to other electromagnetic waves with different energy ranges (e.g. visible light, infrared light, etc.), they are reflected and refracted at the interface of two kinds of medium with different absorption qualities. The characteristics of the phenomena occurring between the X-rays and the medium depend on the physical and chemical composition of the material and on the energy of the electromagnetic radiation. Of the interactions between X-rays and matter, three types play an important role: the photoelectric effect, the inelastic scattering (the Compton scattering), and the elastic scattering (the Thomson scattering and the Rayleigh scattering).
During the photoelectric effect the X-ray photon transfers its total energy to one of the bound electrons of an atom, which thus becomes a free electron, or gets to a bound state with a lower binding energy. As a result, an electron deficiency (co-called vacancy) is created in the electron shell, i.e. the atom gets excited (the total energy of the atom will be higher compared to the energy of the ground state). The so-called photoelectron, which is emitted from the atom, loses its kinetic energy during a series of interactions within the material and is captured again. If the phenomenon takes place close to the surface of the material, then the photoelectron is likely to leave the medium by which it has been emitted. The electron-deficient state of the material can be terminated by two processes:

The vacancy can be filled by an electron from a higher-energy shell, which results in the emission of a photon, the energy of which corresponds to the energy difference between the two shells. This atomic phenomenon is called X-ray fluorescence and the emitted photon is called characteristic photon. The energy of the inner electron shells of the chemical elements is such that this process results in the emission of X-rays. The main steps of the process are shown in Figure 1., in which the horizontal lines symbolize the binding energies of the individual electronic states.

Figure 1. The photoelectric effect and the emission of the characteristic X-radiation

During the other possible way of the atomic de-excitation a radiation-free transition is realized. In this case the change in the potential energy of the electron filling the electron-deficient state is not followed by the emission of a photon, but by the emission of an atomic electron (Auger effect). The energy released during the de-excitation covers the binding energy of the Auger electron as well as the kinetic energy of the electron freed from its bound atomic state.

There is a clear mathematical relationship between the energy of the characteristic X-radiation and the atomic number of the emitting atom, which is called Moseley’s law:

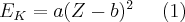
$E_K=a(Z-b)^{2} \;\;\;\;\; (1)$

where Z is the atomic number of the atom, E_K is the energy of the K shell, and a and b are constants. The relationship between the atomic number of an atom and the characteristic X-rays it emits makes it possible to identify an unknown element in a material.

The two possible ways of deexcitation (the characteristic emission of X-rays and the Auger phenomenon) following the photoelectric effect are competing processes and the probability of their occurrence is dependent on the atomic number and the quantum number of the electron shell. The ratio of the probability of the two types of processes can be characterized by the fluorescence yield:

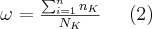
$\omega = \frac{\sum_{i=1}^{n}n_K}{N_K} \;\;\;\;\; (2)$

where 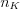n_K is the number of the electrons de-excited from the different L, M, N, etc. shells to the K shell per unit time, while 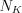N_K is the number of K-shell vacancies created per unit time. Of course, the fluorescence yield, the value of which increases monotonously with the atomic number, can be defined in a similar way for the other shells as well. The theoretical model of the phenomenon was created by Albert Einstein in 1905, for which he received a Nobel Prize in 1921. Although the photoelectric effect is used in several material testing methods to determine the different characteristics of the materials, it does not play a significant role in medical imaging. In the atoms of the living tissue the electrons in the K shells are weakly bound (<500 eV), because the atomic number of most atoms here is lower than 9. Since the absorption of the photons belonging to this energy range is high, the mean free path is in the order of magnitude of a single cell (e.g. E=1 keV, in muscle tissue approximately 2.7 μm). This means that the characteristic photons, which are virtually perfectly absorbed over short distances, do not have to be taken into consideration in X-ray diagnostic imaging, since these photons do not reach the detector. The only chemical element which emits higher-energy photons and is present in larger quantities in certain tissues is calcium, the energy of the characteristic photons of which is in the energy range of 3.6-4.1 keV.

The cross section of the photoelectric effect is dependent on the atomic number of the absorbing medium and the energy of the X-ray beam. The probability of the photoelectric effect at a given photon energy is approximately proportional to the cube of the atomic number of the absorbing medium (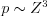 $p \sim Z^{3}$ ), while in the case of a specific element it is inversely proportional to the cube of the energy ( 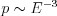$p \sim E^{-3}$ ). This strong dependence on the atomic number plays a significant role during diagnostic imaging, since, due to the differences in the absorption properties of the different tissues, the small differences in the atomic numbers of the individual tissue types give well detectable contrast as well. In addition, it follows from the strong non-linear energy-dependence that in the case of the absorption of the X-rays, primarily the lower energy ranges contribute to the distinguishability of the different tissue types.

Other types of interactions can also occur between the atoms and the electrons of matter and the X-ray photons: e.g. elastic scattering (also known as Rayleigh scattering), or inelastic scattering (also known as Compton scattering).

The elastic (or coherent) scattering process was first modelled by Thomson, who applied classical electrodynamics 1. The expression “elastic” refers to the fact that the photon emitted during the interaction has the total energy of the incoming photon, which means that similarly to elastic mechanical collisions, there is no energy dissipation during the process and the total incoming energy is carried away by the scattered photon. The phenomenon described by Thomson (Thomson scattering) occurs between a non-polarized electromagnetic beam (optical, X-ray or gamma radiation) and a free electron. The electromagnetic field interacting with the free electron causes the electron to harmonically oscillate. The oscillating electron produces a time-varying electromagnetic field with a polar pattern corresponding to the direction of the oscillation, i.e. to the directional distribution typical of dipole radiation 2. Based on this simple model, the angle-dependent intensity distribution of Thomson scattering can be calculated, as well as the angle-dependent differential cross section of the process, which can be expressed by formulae (3). This scattering phenomenon is significant at low energies and at energies far from the atomic resonance energies, i.e. if the condition 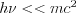$h\nu << mc^{2}$ for the frequency of the photon is met. The first formula provides the angle-dependent cross section, i.e. the proportion of energy emitted per unit solid angle.

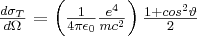
$\frac{d\sigma_T}{d\Omega }=\left ( \frac{1}{4\pi\epsilon_0} \frac{e^{4}}{mc^{2}} \right ) \frac{1+cos^{2}\vartheta }{2}$

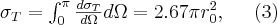
$\sigma_T=\int_{0}^{\pi}\frac{d\sigma_T}{d\Omega } d\Omega=2.67\pi r_0^{2}, \;\;\;\;\; (3)$

where 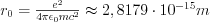$r_0=\frac{e^{2}}{4\pi\epsilon_0 mc^{2}} \approx 2,8179 \cdot 10^{-15}m$ is the so-called classical electron radius, 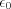$\epsilon_0$ is the relative permittivity of vacuum, c is the speed of light, e and m are the charge and the mass of the electron, r is the distance of the scattering object and the place of detection of the scattered radiation, and 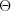$\Theta$ is the angle enclosed by the direction of propagation of the incoming radiation and the scattered radiation. The cross section does not depend on the energy of the primary photon.
The nature of the scattering and the angle distribution of the cross section change if we presume that the scattering electron is harmonically bound and if we take into consideration the effect of every (bound) electron of an atom on the scattering process. This type of scattering, when an electromagnetic interaction takes place between a photon and one or more bound atomic electrons in a way that the photon does not ionize or excite the atom, is called Rayleigh scattering. At the end of the process (similarly to Thomson scattering) a photon, the energy of which is equal to the energy of the photon interacting with the atom, leaves the atom, i.e. the frequency of the incoming and the outgoing photons is equivalent. It can again be presumed that the electrons are forced to oscillate as a result of the external electromagnetic field (X-ray or gamma radiation) 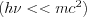$(h\nu << mc^{2})$ . The frequency of the exciting electric field is significantly higher in the case of X-ray and gamma radiation than the natural frequency of the weakly bound atomic electrons. As a consequence, in an opposite phase of the electric field of the exciting photon as compared to the oscillating electron, the electron will decelerate and emit electromagnetic radiation. If the excitation frequency is significantly lower than the natural frequency of the atomic electrons (i.e. the frequency of the absorption edges), then the atom emits electromagnetic radiation, the frequency of which is equivalent to that of the radiation initiating the process, i.e. the two rays will be coherent with each other. Since the bound atomic electrons cannot be considered perfectly independent of each other due to the Coulomb interaction, the intensity of the emitted radiation cannot be produced as the simple algebraic sum of the radiation contribution of the individual electrons. The combined effect of the atomic electrons can be taken into consideration when calculating the cross section by using a so-called scattering factor 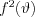$f^{2}(\vartheta)$ , as expressed in formula (4):

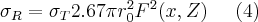
$\sigma_R = \sigma_T 2.67\pi r_{0}^{2}F^{2} (x,Z) \;\;\;\;\; (4)$

where 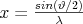$x=\frac{sin(\vartheta /2)}{\lambda}$ is the momentum, λ is the wavelength of the incoming photon, Z is the atomic number of the atoms of the scattering medium, and F(x,Z) is the angle-dependent atomic form factor. This last factor can be used to take into account the effect all atomic electrons have on the cross section. Formula (4) shows that the cross section of Rayleigh scattering is dependent on the energy of the exciting photon, as well as on the atomic number of the atom which interacts with it. The cross section of Rayleigh scattering is usually much lower than that of other interactions between atoms and photons (photoelectric effect, Compton scattering), still, this atomic phenomenon provides the basis for the measurement procedures based on X-ray diffraction and Bragg reflection.

In contrast to the elastic scattering processes, the photon transfers only a small part of its energy to the atomic electron during Compton scattering 3. This phenomenon is only realized if the binding energy of the electron is little compared to the energy of the photon. Such electrons can be found in the outer shells of the atoms. If the binding energy of the electron is negligible compared to the energy of the photon, then the momentum transfer between the photon and the electron becomes possible. During the process, the energy and the direction of propagation of the incoming photon change, while the interacting electron becomes free. The mathematical relationship between the magnitude of the energy of the primary photon (E) and the scattered photon (E*) is expressed by formula (5), where 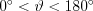$0^{\circ}<\vartheta<180^{\circ}$ is the angle enclosed by the direction of propagation of the scattered photon and the direction of the primary photon, m is the rest mass of the electron, and c is the speed of light.

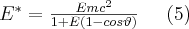
$E^{*}=\frac{Emc^{2}}{1+E(1-cos\vartheta)} \;\;\;\;\; (5)$

In 1922, O. Klein and Y. Nishina were the first to calculate the angle-dependent cross section of the Compton process, which was compared to the direction of propagation of the primary photon and was determined for a unit solid angle. Expression (6) is known as the Klein-Nishina formula:

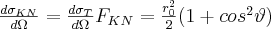
$\frac{d\sigma_{KN}}{d\Omega}=\frac{d\sigma_T}{d\Omega}F_{KN}=\frac{r_0^{2}}{2}(1+cos^{2}\vartheta)$

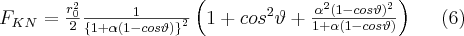
$F_{KN}=\frac{r_0^{2}}{2}\frac{1}{\left \{ 1+\alpha(1-cos\vartheta) \right \}^{2}}\left ( 1+cos^{2}\vartheta+\frac{\alpha^{2}(1-cos\vartheta)^{2}}{1+\alpha(1-cos\vartheta)} \right ) \;\;\;\;\;(6)$

where F_KN is the Klein-Nishina form factor, 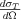$\frac{d\sigma_{T}}{d\Omega}$ is the Thomson cross section given in formula (3), and 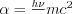$\alpha=\frac{h\nu}{}mc^{2}$ . The angular distribution of the differential cross section of the Compton process is depicted in Figure 2. at the different values of the α parameter. The Thomson scattering by a free electron corresponds to 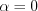$\alpha=0$ It can be noticed that the probability of forward scattering increases significantly at high photon energies.
Depending on the value of angle 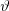$\vartheta$ the energy of the electron pushed during the Compton scattering process falls in a well-defined energy range. A direct consequence of this is that a Compton background with continuous energy distribution can always be observed in X-ray and gamma-ray spectra.
In the case of high-energy electromagnetic radiation (the energy of which is out of the X-ray energy range), pair production can occur. During this process, an electron-positron pair can be formed from a gamma photon of appropriate energy in the electric field of an atomic nucleus of the detector material. For the pair production to occur, the energy of the photon must be higher than 2mc² =1.022 MeV. If the condition 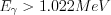$E_{\gamma}>1.022MeV$ is met, i.e. the energy of the photon is higher than the sum of the rest masses of the two particles, then the remaining energy of the photon is spent on the kinetic energy of the electron and the positron.

Figure 2. The angle-dependent cross section of the Compton process for different photon energies

The positron later combines with an electron resulting in annihilation, during which two 0.511 MeV photons appear at nearly 180 degrees. The cross section of the pair production process is proportional to expression (7):

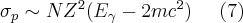
$\sigma_p \sim NZ^{2}(E_{\gamma}-2mc^{2})\;\;\;\;\; (7)$

If a gamma or an X-ray beam traverses a layer of matter, as a result of the processes discussed above the intensity of the beams decreases and scattered photons appear.

Absorption

If a parallel beam of X-rays enters a slab of material of thickness x, the intesity of the beam will decrease as described by (8).

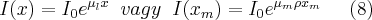
$I(x)=I_0 e^{\mu_l x} \; \; vagy \; \; I(x_m)=I_0 e^{\mu_m \rho x_m} \;\;\;\;\; (8)$

Here I₀ is the netering beam intensity (a quantity proportional to the energy flow density of the radiation), I(x) is the intensity of the beam exiting the sample of thickness x , µ_l(1/cm) and µ_m(cm²/g) are the so called linear and mass absorption coefficients, 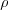$\rho$ is the density of the material and x_l(cm) and x_m(g/cm²) is the thickness of the absorbing material.
In formula (8) the thickness of the absorbing layer can be given both in (1/cm or g/cm²) units, depending on which absorption definition we use. The practical advantage of the use of mass absorption coefficient is that in this case one need not know the density of the material , only the mass of the layer behind a unit area.

The reason for the decrease of intensity is the above detailed interaction phenomena between the photons and atoms, ie. photoelectric effect, coherent and incoherent scatter etc. The mass absorption coecfficient and the linear absorption coefficients are related by 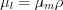$\mu_l=\mu_m\rho$ , from which it is obvious that the liner absorption coefficient depends on the density of the material. Since the beam intensity is reduced due to all of the three interactions depending o the energy of the X-rays and the Z-number of the medium, the mass absorption coefficient can be witten as the sum of three coefficients:

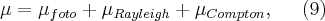
$\mu=\mu_{foto}+\mu_{Rayleigh}+\mu_{Compton}, \;\;\;\;\; (9)$

where the coefficients denote the mass absorption coefficients corresponding to the different processes. Fig. 3 shows an example of the energy dependence of the three phenomena, where the absorption functions of lead can be seen as a function of energy for the energy range 1< E < 200 keV.
In general we can state that the value of the absorption coefficient is a relatively complicated function of the energy of of the radiation (Fig. 3), which is mostly related to ionization cross section of the individual electron shells (photoelectric effect) since in the X-ray energy region the vast majority of absorption is due to this process. One can observe "jumps" in the absorption functions at well determined energies, which are characteristic of the given element. These are called absorption edges. The energy of an edge corresponds to the ionization energy of an atomic shell.

Figure 3

Formula (8), which describes the absorption, can also be obtained by theoretical considerations. Let us assume that all of the atoms present in the absorbing layer contribute to the reduction process. If the cross section of a single atom is , then the absorbing effect of all of the atoms present in the layer is described by (10):

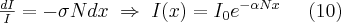
$\frac{dI}{I}=-\sigma N dx \; \Rightarrow \; I(x)=I_0 e^{-\alpha Nx}\;\;\;\;\; (10)$

where is the density of the atoms in the absorbing layer (atom/cm³) and I₀ is the intensity of radiation entering the medium. Comparing formulae (8) and (10), the following functions are obtained for the absorption definition:

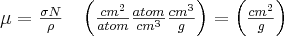
$\mu=\frac{\sigma N}{\rho} \;\;\; \left ( \frac{cm^{2}}{atom} \frac{atom}{cm^{3}} \frac{cm^{3}}{g}\right )= \left ( \frac{cm^{2}}{g} \right)$

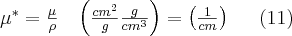
$\mu^{*}=\frac{\mu}{\rho} \;\;\; \left ( \frac{cm^{2}}{g} \frac{g}{cm^{3}} \right )= \left ( \frac{1}{cm} \right) \;\;\;\;\; (11)$

If the absorbing material containes more than one chemical element in the form of compounds or mixtures, this fact must be accounted for by using the following equation:

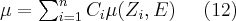
$\mu=\sum_{i=1}^{n} C_i \mu(Z_i,E) \;\;\;\;\; (12)$

where i=1,...,n is the index of chemical elements in the sample, C_i is the mass concentration of the az ith element and µ(Z_i,E) is the atomic number and X-ray energy dependent mass absorption coefficient of the ith element. Obviously, the condition 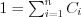$1=\sum_{i=1}^{n} C_i$ must in all cases be met.

If the energy of an X-ray photon is larger than that of an absorption edge, the degree of absorption will be significantly larger than for photons having lower energy than that of the absorption edge. Independent of the edges, the absorption in the X-ray range shows a monotonously decreasing tendency as the energy increases.
The contribution of photoelectric effect to the absorption and the energy and atomic number dependence between two consecutive edges can be described by the so called Bragg–Pierce empirical formula:

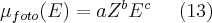
$\mu_{foto}(E)=aZ^{b}E^{c} \;\;\;\;\; (13)$

where 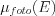$\mu_{foto}(E)$ is the value of absorption at energy E, Z is the atomic number, while the value of the coefficients a, b and c changes in the ranges between the absorption edges. The energy dependent absorption of the elements and compound materials can be found a a number of publications. Also, there are tables available directly for practical usage containing the absorption values. The homepage of NIST (National Institute of Standard and Technology) contains such a comprehensive absorption data as a function of the energy of the electromagnetic wave (frequency) for all of the elements of the periodic table and also for some frequently occuring material compounds and various human tissue types. (http://www.nist.gov/pml/data/index.cfm)

Concerning the absorption of X-rays a few definitions and concepts are introduced:

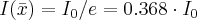
The average mean free path of photons: $I(\bar{x})=I_0/e=0.368 \cdot I_0$
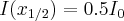
Half-value layer: $I(x_{1/2})=0.5I_0$
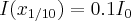
Tenth-value layer: $I(x_{1/10})=0.1I_0$

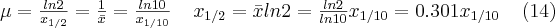
$\mu=\frac{ln2}{x_{1/2}}=\frac{1}{\bar{x}}=\frac{ln10}{x_{1/10}} \;\;\;\; x_{1/2}=\bar{x} ln2 = \frac{ln2}{ln10} x_{1/10}=0.301x_{1/10} \;\;\;\; (14)$

In Table 1 we summarize the most important properties of the photon-electron and photon-atom phenomena, giving the predominant energy ranges, where the given process prevails in the photon-particle interactions. The table also shows the type of particle produced and the Z- and energy-dependence of the given process.

Table 1. Comparison of properties of photon-electron phenomena

Phenomenon	Photoelectric effect	Rayleigh-scattering	Compton-effect	Pair-interaction
Characteristics	photon dies	scattering	scattering	photon dies
Z dependence of mass absorption	µ∝Z⁴	µ∝Z²	µ∝Z	µ∝Z
cInduced particle	photoelektron	-----	Compton electron	electron-positron pair
Characteristic energy range	<20keV	<20keV	20keV-10MeV	10MeV<
Characteristic energy range in water	< 500 keV	< 100 keV	500 keV – 3 MeV	3 MeV <

Figure 4

Besides the mass absorption and linear absorption functions there are other functions that describe absorption properties. These can be related to a basic process or atomic phenomenon.

Atomic cross section (µ_a, cm²/atom)
Electric cross section (µ_e, cm²/elektron)
Energy transfer coefficient (µ_TR)
Energy-absorption function (µ_ab)

The below (15) formulae give the relationship between the above quantities:

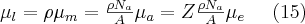
$\mu_l=\rho\mu_m=\frac{\rho N_a}{A}\mu_a=Z\frac{\rho N_a}{A}\mu_e \;\;\;\;\; (15)$

The energy transfer absorption (µ_TR) tells the amount of energy transferred by the photon to the (charged) particles taking part in the photon-atom processes, while the energy-absorption function describes the total amount of energy the photon transfers to the medium. Accordingly, the following definitions can be written for these quantities:

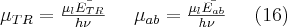
$\mu_{TR}=\frac{\mu_l \bar{E_{TR}}}{h \nu} \;\;\;\;\; \mu_{ab}=\frac{\mu_l \bar{E_{ab}}}{h \nu} \;\;\;\;\; (16)$

where Ē_TR is the average energy that a photon transfers to the created charged particles, while Ē_ab describes how much energy the photon has transferred to the absorbing medium on the average. The relationship between the above defined average energy values is given by (17):

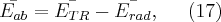
$\bar{E_{ab}}=\bar{E_{TR}}-\bar{E_{rad}}, \;\;\;\;\; (17)$

where Ē_rad is the average energy lost by particles in collisions and electromagnetic interactions which imply some type of electromagnetic radiaion. Accordingly, the following equation holds for these absorption coefficients:

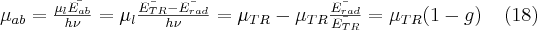
$\mu_{ab}=\frac{\mu_l \bar{E_{ab}}}{h \nu}=\mu_l \frac{\bar{E_{TR}}-\bar{E_{rad}}}{h \nu}=\mu_{TR}-\mu_{TR} \frac{\bar{E_{rad}}}{\bar{E_{TR}}}=\mu_{TR} (1-g) \;\;\;\; (18)$

where the factor g is the portion of energy that is dissipated by radiation in the medium (radiative fraction). Note here that most of the energy transferred to the medium goes away by Bremsstrahlung (see below).

In diagnostic examinations one of the most important design aspect is the minimalization of the patient dose parallel with the maximization of the information obtained from the examined area. In this respect it is important that the energy absorbed in tissues should be as low as possible. In order to measure this, the notion of linear energy transfer (LET) was introduced. It tells the specific energy (per unit length) transferred by the particle along its path in the medium in ionization processes. The high-LET particles are the alpha-particle, ions, proton etc., the absorption of which in living tissues cause more serious harm. Significantly less energy is transferred when light particles (electron or positron) or electromagnetic waves are absorbed in the medium.

The emission and properties of Bremsstrahlung

If a charged particle is decelerated in an electric or magnetic field (changes the magnitude or direction of its velocity), electromagnetic radiation is induced. This radiation has a continuous spectrum (the frequency or wavelength distribution is continuous) and the electric and magnetic field stregth vectors are perpendicular to the direction of travel (transversal wave). The name Bremsstrahlung refers to the German discovery.
In order to understand and describe the phenomenon, let us examine the electric field of a particle at rest (Fig. 5a) and another one that moves at a constant speed in a straight line (Fig. 5b). According to Fig. 5, the static electric field of a particle at rest having a charge Q is spherically symmetric, ie. the components by the two coordinate axes can be described by equation (19):

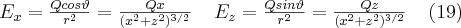
$E_x=\frac{Q cos{\vartheta}}{r^{2}}=\frac{Qx}{(x^{2}+z^{2})^{3/2}} \;\;\;\; E_z=\frac{Q sin{\vartheta}}{r^{2}}=\frac{Qz}{(x^{2}+z^{2})^{3/2}} \;\;\;\; (19)$

If a particle is moving at a constant speed v relative to the observer (or if the observer is moving relative to the particle), the structure of the electric field seen by the observer changes compared to the static case. In order to calculate the structure of the electric field one has to account for the Lorentzs transforation between the two frames of reference. Let us switch to spherical coordinates:

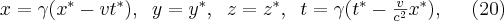
$x=\gamma (x^{*}-vt^{*}), \;\; y=y^{*}, \;\; z=z^{*}, \;\; t=\gamma (t^{*}-\frac{v}{c^{2}}x^{*}), \;\;\;\;\; (20)$

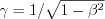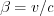where $\gamma=1/\sqrt{1-\beta^{2}}$ and $\beta=v/c$ , c is the speed of light. Thus the electric field generated by a point charge in the (*) frame of reference can be given by equation (20)

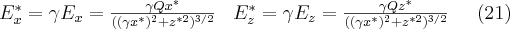
$E_{x}^{*}=\gamma E_x=\frac{\gamma Qx^{*}}{((\gamma x^{*})^{2}+z^{*2})^{3/2}} \;\;\; E_{z}^{*}=\gamma E_z=\frac{\gamma Qz^{*}}{((\gamma x^{*})^{2}+z^{*2})^{3/2}} \;\;\;\;\; (21)$

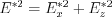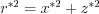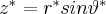The angular distribution of the field strength $E^{*2}=E_{x}^{*2}+E_{z}^{*2}$ in a stationary and moving frame of reference (with the aid of equations $r^{*2}=x^{*2}+z^{*2}$ and $z^{*}=r^{*}sin \vartheta^{*}$ ) can be described with the following function:

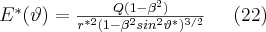
$E^{*}(\vartheta)= \frac{Q(1-\beta^{2})}{r^{*2}(1-\beta^{2}sin^{2}\vartheta^{*})^{3/2}}\;\;\;\;\; (22)$

If the speed is non-relativistic, that is 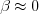$\beta \approx 0$ , then we obtain the electric field of the stationary charge. However, if 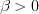$\beta > 0$ , the structure of the electric field is not isotropic according to function (22) in contrast to the field of the stationary charge. Accordingly, the difference between the two space structures in the stationary and moving (relative to the observer) frame of reference is depicted in Fig. 5.

Figure 5 The electric field of a positively charged particle in case it is at rest (a) and in case it moves at velocity v in direction x (b)

We may conclude that in a moving frame of reference the space ceases to be isotropic, which is characteristic of the stationary frame. Accordingly, the field strength will not vary as predicted by 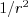$1/r^{2}$ .

Figure 6

If we look at the electric field of an accelerating point charge as a function of time, we obtain an anisotropic field instead of the static electric field of a stationary charge. The change in the structure of the field (isotropic-anisotropic) propagates in space at the speed of light.
After the charge stops accelerating, the structure of the field will be that of a charge moving at 0>Δv (Figure 6). It can be calculated that in the transition range (isotropic-anisotropic transition) the magnitude and directional distribution of the electric vector of the field depends on the magnitude and duration of the acceleration.
Similar conclusions can be drawn on the reverse case, when a charge moving at speed v decelerates and then stops (Fig. 7). One can observe from Figs 6 and 7 that in the transition range the direction of the field strength is approximately tangential and the direction of travel is almost radial. This means that the region that moves at the speed of light behaves as a transversal wave.

Figure 7

This type of electromagnetic radiation may be induced by an electron oscillating in a dipole or by an electron that decelerates in the electric field of atoms. By applying this principle, one can create radio waves of different wavelengths, certain EM waves in the optical range and also breaking radiation, ie. Bremsstrahlung.

Characteristic X-rays

If a vacancy is created on one of the energy levels of the electron shell of an atom, that will be filled by an electron which has a higher energy level (principal quantum number). The higher the atomic number, the larger the number of different deexcitations. Nevertheless, only those transitions may occur which satisfy all the conservation principles. Therefore, many combinations can not occur at all. These are called forbidden transitions.

Figure 8

Of the very large number of possibilities the one will occur most of the time that has the highest transition probability and that is not forbidden by the selection rules of quantum mechanics. This fact can be experienced during spectroscopy in a way that the intensity ratios in characteristic X-ray spectra constitute a system of well determined values which correspond to a given element. Fig. 8 shows the energy level structure and possible transitions of an atom (the figure actually shows the level structure of copper).
The most probable transition is the so called electric dipole transition. Therefore, the quadruple transitions result in significantly smaller intenstity peaks in X-ray spectra. The characteristic lines corresponding to dipole and quadruple transitions are called diagram lines. The relevant selection rules are as follows:

In a dipole transition the principal quantum number can change arbitrarily, with the exception of zero.
The azimuthal quantum number can change Δl = ±1, while for the magnetic quantum number Δj = 0, ±1

In the case of a quadruple transition Δn ≠ 0, Δl = ±2, Δj = 0, ±1, ±2.

Originally different systems were developed for the notation of characteristic X-ray lines. The notations have been rationalized in the last 20 years taking into account the up-to-date X-ray spectroscopy techniques and principles.

Table 3. Notation of X-ray lines in the Siegbahn system and the system suggested by IUPAC

Siegbahn	IUPAC	Siegbahn	IUPAC	Siegbahn	IUPAC	Siegbahn	IUPAC
Kα1	K-L3	Lα1	L3-M5	Lγ1	L2-N4	Mα1	M5-N7
Kα2	K-L2	Lα2	L3-M4	Lγ2	L1-N2	Mα2	M5-N6
Kβ1	K-M3	Lβ1	L2-M4	Lγ3	L1-N3	Mβ	M4-N6
KIβ2	K-N3	Lβ2	L3-N5	Lγ4	L1-O3	Mγ	M3-N5
KIIβ2	K-N2	Lβ3	L1-M3	Lγ4'	L1-O2	Mζ	M4,5-N2,3
Kβ3	K-M2	Lβ4	L1-M2	Lγ5	L2-N1
KIβ4	K-N5	Lβ5	L3-O4,5	Lγ6	L2-O4
KIIβ4	K-N4	Lβ6	L3-N1	Lγ8	L2-O1
Kβ4x	K-N4	Lβ7	L3-O1	Lγ8'	L2-N6(7)
KIβ5	K-M5	Lβ7'	L3-N6,7	Lη	L2-M1
KIIβ5	K-M4	Lβ9	L1-M5	Ll	L3-M1
		Lβ10	L1-M4	Ls	L3-M3
		Lβ15	L3-N4	Lt	L3-M2
		Lβ17	L2-M3	Lu	L3-N6,7
				Lv	L2-N6(7)

In the early days of X-ray spectroscopy the so called Siegbahn system was used. Although it contained almost all of the possible transitions, there were quite a number of unreasonable traits. In Table 3 one can see the Siegbahn notation corresponding to the different lines, along with the notation suggested by IUPAC, which is more reasonable and applicable to modern technologies. The latter denotes the lines in accordance with their origin, with the aid of the energy levels of the transition. In this way the user can directly see what transition the given line belongs to.

The lines denoted in this way are called diagram lines. In real X-ray spectra other lines may be present (satelite lines), which are impossible to describe using this system. The electron orbits are denoted by the letters K, L, M, N (n=1: K, n=2: L, n=3: M,…). Figure 8 is distorted in terms of the ratio of the energy of the levels, since in reality the difference between the energy levels belonging to a given principal quantum number is significantly smaller. The system of notation for the classification of characteristic X-rays is the following: if the transition occurs to the K (L,M, etc.) shell, we talk about radiation in the K (L,M, etc.) series, while the most intense line within one series is the α. Eg. in the case of the K series the L → K transition corresponds to α ( Kα), while the line corresponding to the M → K transition is called the Kβ line. Within one subseries the lines corresponding to the fine structure are denoted by numbered subscripts.

Satelite lines may be produced if the ionizing effect upon the atom is so strong that the probability of the multiple ionization of a single atom is not negligible. The energy of these lines is a little different from that of the diagram lines. The energy line level structure of a multiply ionized atom is somewhat shifted compared to an atom with single ionization since the number of charges constituting the atom changes. Therefore, the energy differences corresponding to the transitions change. This phenomenon is observable in the X-ray spectrum as the presence of "new" lines, which are shifted compared to the diagram lines.

Fig. 9 shows a typical X-ray spectrum that contains characteristic lines. The object of this spectum is a steel alloy, which contains a number of constituents of different concentrations.

Figure 9

The spectrum was recorded in the following way. The steel sample was excited by photoelectric effect induced by Bremsstrahlung generated from an X-ray tube, which has a continous energy distribution. The vacancies created were filled by electrons of higher principal quantum numbers, which resulted in the emission of characteristic lines of of different intensity. Besides that, the intensity of the individual characteristic lines depends on the concentration of the given element in the sample and the atomic number of the constituents of the sample through the Z-dependence of absorption. Therefore, in accordance with the element concentrations and the electron level trasition probabilities, the intensity of the characteristic X-ray lines are different. This fact can be used to determine the element composition of an unknown sample. This method is called X-ray flourescens analysis.

Polarization of X-rays

X-rays are transversal waves so they may exist in polarized form. When a dipole oscillator radiates, the electric vector of the of the EM waves will be parallel to the direction of the oscillation of the charges constituting the dipole and will be perpendicular to the direction of travel. It might as well happen that not all of the rays are polarized in a beam of X-rays. In this case the beam is called partially polarized. The measure of polarization is given by (23):

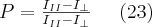
$P=\frac{I_{II}-I_{\perp} }{I_{II}-I_{\perp}} \;\;\;\;\; (23)$

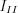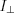where $I_{II}$ and $I_{\perp}$ are the intensity of the waves parallel and perpendicular to the plane of emission. If the X-ray beam is fully polarized, ie. P=1, the electric vector of the entire beam is parallel to the plane of emission. However, if any two directions being perpendicular to each other have the same intensity, the beam is called unpolarized (P=0).
Since the scattering processes and their cross sections depend on the degree of polarization of the X-ray beam, this characteristics has a major role in X-ray spectroscopy and in X-ray based imaging methods. The Bremsstrahlung created by X-ray tubes is partially polarized, the degree of which depends on the construction, geometry and implementation of the X-ray source.
Fig. 10 shows the change in the degree of polarization. Here the quantity defined by (23) is depicted as a function of X-ray energy, tube voltage and atomic number of the anode 2. The frequency value is the maximal frequency present in the spectrum of the X-ray tube (short-wave limit), which is determined by the anode-cathode voltage switched on the X-ray tube (see next section). According to Fig. 10 the degree of polarization is almost 1 near the short-wave limit, while the beam is totally unpolarized in the range of lower frequencies.

10. ábra

The original document is available at http://537999.nhjqzg.asia/tiki-index.php?page=The+Fundamental+Interactions+between+X-rays+and+Matter