Laplace transformation of characteristic and any other typical functions

Laplace transformation of several important functions (characteristic and typical) will be provided in the followings and they can be proofed by the earlier used methods.

a) Dirac-delta 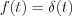$ f(t)=\delta(t)

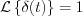
$ \mathcal{L} \left \{ \delta(t) \right \}= 1

b) Heavyside unit step function 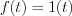$ f(t)=1(t)

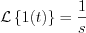
$ \mathcal{L} \left \{ 1(t) \right \}= \frac{1}{s}

c) Power function 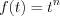$ f(t)=t^n

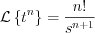
$ \mathcal{L} \left \{ t^n \right \}= \frac{n!}{s^{n+1}}

d.) Exponential function 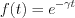$f(t) = e^{-\gamma t}, where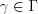 $\gamma \in \Gamma

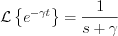
$\mathcal{L}\le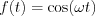ft \{e^{-\gamma t}\right \} = \frac{1}{s+\gamma}

e.) $f(t) = \text{cos}(\omega t) i.e. the simple harmonic oscillator

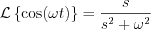
$\mathcal{L}\left \{\text{cos}(\omega t)\right \} = \frac{s}{s^{2}+\omega^{2}}

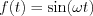f.) $f(t)=\text{sin}(\omega t) i.e. the simple harmonic oscillator

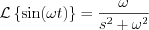
$\mathcal{L}\left\{ \text{sin}(\omega t)\right \} = \frac{\omega}{s^{2} + \omega^{2}}

g.) Laplace transformation of periodic step function. See the following two figures (Figure 29., 30.).

Image
Figure 29.

 

Image
Figure 30.

 
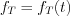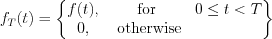
The first single periodic of $f(t) periodic step function is denoted by $f_{T} = f_{T}(t), where $f_{T}(t) =\begin{Bmatrix} f(t), & \text{ for } & 0 \leq t<T\\ 0, & \text{ otherwise} \end{Bmatrix}

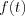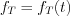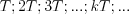$f(t) periodic function presented on the Figure 29. is possible to build up by the superposition of the single periodic $f_{T} = f_{T}(t) function with shifting $T; 2T; 3T; ... ;kT; ....
The analytical expression is the following:

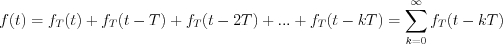
$f(t) = f_{T}(t)+f_{T}(t-T)+f_{T}(t-2T)+...+f_{T}(t-kT) = \sum_{k=0}^{\infty}f_{T}(t-kT)

Execute the Laplace transformation by the shifting theorem and the superposition rule.

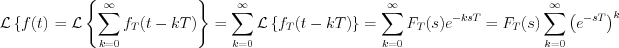
$\mathcal{L}\left \{f(t)\right} = \mathcal{L}\left \{ \sum_{k=0}^{\infty}f_{T}(t-kT)\right \} = \sum_{k=0}^{\infty}\mathcal{L}\left \{f_{T}(t-kT)\right \} = \sum_{k=0}^{\infty}F_{T}(s)e^{-ksT} = F_{T}(s)\sum_{k=0}^{\infty}\left(e^{-sT}\right) ^{k}

 
It is possible to see, that 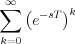$\sum_{k=0}^{\infty}\left( e^{-sT}\right)^{k} is an infinite geometrical series, where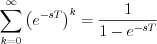 $\sum_{k=0}^{\infty}\left(e^{-sT}\right)^{k} = \frac{1}{1-e^{-sT}}

Consequently, the Laplace transformation of the periodic step function is:

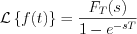$\mathcal{L}\left\{f(t)\right\} = \frac{F_{T}(s)}{1-e^{-sT}}, where

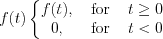
$f(t)\left\{\begin{matrix} f(t), & \text{ for } & t \geq 0\\ 0, & \text{ for } & t<0 \end{matrix}\right.

 
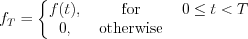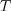$f_{T} = \left\{\begin{matrix} f(t), & \text{ for } & 0 \leq t < T\\ 0, & \text{ otherwise } \end{matrix}\right., and $T is the periodic of the function

 
h.) Convolution

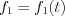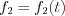Let $f_{1} = f_{1}(t) and $f_{2} = f_{2}(t) be absolutely integrable general step functions as well as their Laplace transformations are:

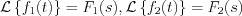
$\mathcal{L}\left\{f_{1} (t) \right\} = F_{1}(s), \mathcal{L}\left\{f_{2}(t)\right\} = F_{2}(s)

Let’s determine the 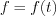$f = f(t) function, if 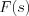$F(s) function in the complex frequency domain is expressed as follow:
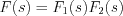
$F(s) = F_{1}(s)F_{2}(s)

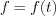$f=f(t) function in the real 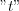$ parameter space can be expressed by the inverse Laplace transformation:

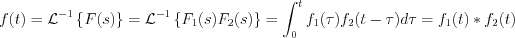
$f(t) = \mathcal{L}^{-1}\left\{F(s)\right\} = \mathcal{L}^{-1}\left\{F_{1}(s)F_{2}(s)\right\} = \int_{0}^{t}f_{1}(\tau)f_{2}(t-\tau)d \tau = f_{1}(t)\ast f_{2}(t)

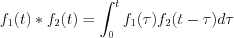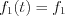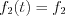The $f_{1}(t) \ast f_{2}(t) = \int_{0}^{t} f_{1}(\tau)f_{2}(t-\tau)d\tau operation is called convolution of $f_{1}(t) = f_{1} and $f_{2}(t)=f_{2} functions.

 
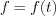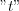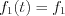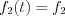Consequently, if a function $f=f(t) in the real $ parameter space can be expressed by $f_{1}(t)=f_{1} and $f_{2}(t) = f_{2} by the following operation:
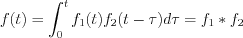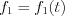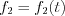$f(t)=\int_{0}^{t}f_{1}(t)f_{2}(t-\tau)d\tau = f_{1} \ast f_{2} , where $f_1=f_1(t) and $f_2=f_2(t) are absolutely integrable, then the result of the transformation into the complex frequency domain is as follow:

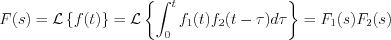$F(s) = \mathcal{L}\left\{f(t)\right\} = \mathcal{L}\left\{ \int_{0}^{t}f_{1}(t)f_{2}(t-\tau)d\tau \right\} = F_{1}(s)F_{2}(s), i.e. the product of the two 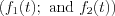$(f_{1}(t); \text{ and }f_{2}(t)) functions in the extended frequency domain:

Let’s see, how can be derived the Laplace transformation of the derivation of a convolution operation.

$\mathcal{L}\left\{\frac{df(t)}{dt}\right\} = \mathcal{L}\left\{\frac{d}{dt}\left[f_{1}(t) \ast f_{2}(t)\right] \right\} = \mathcal{L}\left\{\frac{d}{dt}\int_{0}^{t}f_{1}(t)f_{2}(t-\tau)d\tau \right\}

$\mathcal{L}\left\{ \frac{d}{dt}\left[f_{1}(t) \ast f_{2}(t)\right] \right\} = sF_{1}(s)F_{2}(s)


The original document is available at http://537999.nhjqzg.asia/tiki-index.php?page=Laplace+transformation+of+characteristic+and+any+other+typical+functions